6CCE3EAL: Imagine that we have a solid rod, and we apply heat to the rod: for example, by holding it under a flame. Intuitively, we know that if we hold: Engineering Algorithms Coursework, KCL, UK

Imagine that we have a solid rod, and we apply heat to the rod: for example, by holding it under a flame. Intuitively, we know that if we hold the rod at one end, then eventually we will feel the temperature rising, depending on what the rod is made from: metal rods will heat quickly, whereas a glass rod might not heat at all.

At the molecular level, the ‘hot’ molecules underneath the flame are vibrating rapidly, which causes their adjacent neighbors to also start oscillating. This process repeats, and the further we are from the flame, the more the temperature ‘spread out. If we remove the flame, then the temperature of the rod will eventually decay until it reaches the temperature of the room.

This process is called diffusion. Visually, you can think about adding a small amount of dye to a large container of fluid at rest. Introducing the dye via a syringe at a specific point, we would eventually expect the dye to diffuse amongst the fluid until it can no longer be seen.

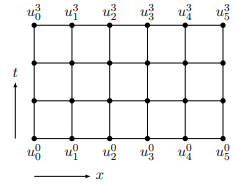
To model this mathematically, consider a solid rod of length L and constant cross-section, and assume that the temperature is constant in each cross-section. Then the temperature can be expressed as a function of both one-dimensional location x, where 0 ≤ x ≤ L and time t ≥ 0. We can therefore express the temperature as a function u(x, y). If the thermal conductivity of the material is given by

a constant α, then we can show that u observes the partial differential equation.

This is called the heat equation.

To solve the heat equation, we also need some additional information, in the same way, that an ODE needs to know an initial value to give a unique answer. In particular, we need to know two things:

• an initial condition: what is the temperature at t = 0?
• the boundary conditions: what is the temperature at x = 0 and x = L?

For this assignment, we will assume the following:

• the initial temperature is given by some known function f(x), so that u(x, 0) = f(x);
• we fix the temperature at each end of the rod, so that u(0, t) = a and u(L, t) = b, where a and b are constants.